IMU Abacus Medal
BLOG: Heidelberg Laureate Forum

One of the prizes awarded to HLF Laureates is the Nevanlinna prize – given every 4 years at the International Congress of Mathematicians, to celebrate outstanding contributions in mathematical aspects of information sciences. That is to say, areas of maths which overlap with computing: complexity theory, logic and programming languages, algorithms, cryptography, pattern recognition, information processing and artificial intelligence, as well as scientific computing and numerical analysis.
This year, the IMU has decided to rename the prize, though it will have the ‘same purpose and scope as the Nevanlinna Prize’. This new award will be called the IMU Abacus Medal, and will be awarded at the International Congress of Mathematicians for the first time in 2022, and will continue to include a cash award of €10,000 and a medal.

The IMU’s press release describes their reason for the choice of this new name:
“The name IMU Abacus Medal relates to the abacus, an ancient device that was used for numerical computations, and it underscores the importance of calculations already in early mathematics. The exact place and time of origin of the abacus is unknown, and it can be considered a truly global artifact associated with mathematics and computation.”
A truly global artifact
The origin of the abacus is indeed unknown, and the word has also been used to describe tablets strewn with sand or sawdust, or covered in wax, used for mathematical calculation. Nowadays, it’s mostly understood to describe some kind of wooden rack of beads which can be used for counting and manipulating numbers. Abacuses have been used for centuries in countries all over the world, but rose to prominence in China and Japan, and were also popular in Europe and Russia.
Babies and small children play with toy abacuses, by sliding beads across to practice counting and learn about numbers – but they are also used by adults as a serious calculation tool, and until fairly recently the use of abacuses was taught in schools – and still commonly is in some places. While it might seem a simple device in this era of electronic calculators and computers, the abacus can perform many of the same functions as a calculator, and a trained operator can perform staggering feats of calculation using a simple rack of beads as a mental aid.
A child’s abacus, like the one pictured above, has ten beads on each bar, which can be slid across to denote the number of hundreds, tens and units in each digit of a number. But some types of calculation abacuses are more streamlined and simple to operate. A soroban, a type of abacus originating in Japan, can be used to add, subtract, multiply and divide numbers, and calculate square and cube roots. It consists of vertical rods each containing four beads in a lower section, and one in an upper section.
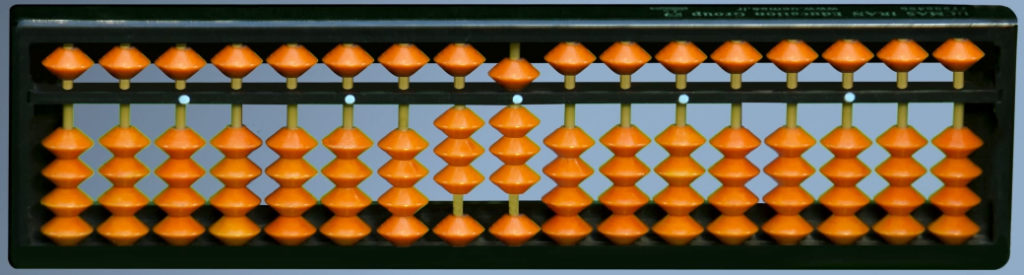
The soroban was developed from an earlier type of abacus from China, called a suanpan, which has two beads above and five below the divider – this still can be used for normal calculations in base ten, by leaving the top and bottom beads in place, but also in hexadecimal, which traditional Chinese units of weight were based on.
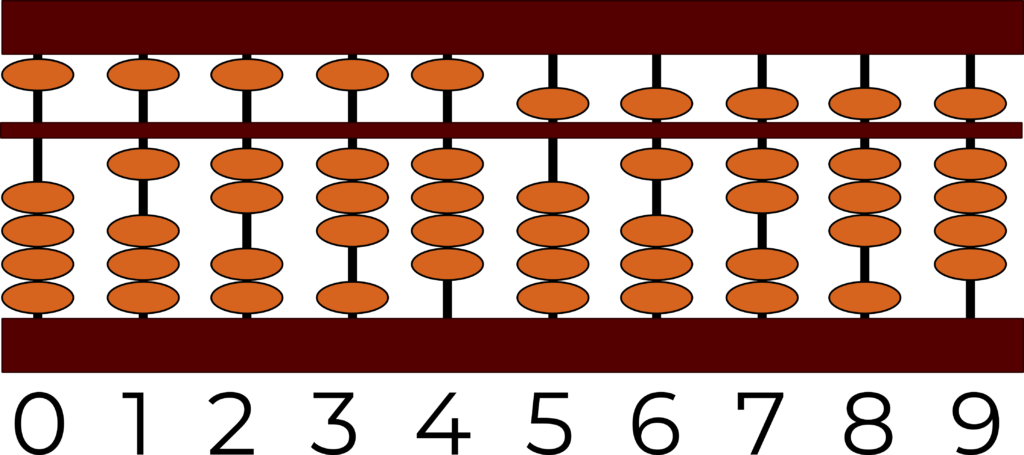
Mechanical Calculator
The 4+1 system of beads can display any number by an arrangement of beads, with each digit of the number corresponding to one column of beads. The number of beads pushed up to the divider could be 1, 2, 3 or 4; the bead above the divider represents a 5, and if it’s pushed down to the bar it means add 5 to whatever’s below. The digits 0-9 are represented as shown:
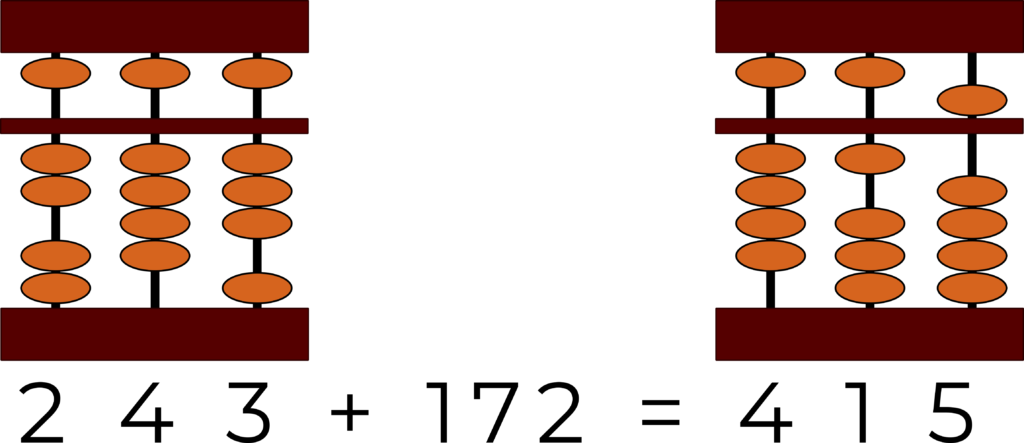
Addition is performed digit-by-digit, in the same way you would do it using a pen and paper. For example, if you’re adding together two numbers on paper, you first add the right-hand digits together and write the result in the right hand column; if the result is more than 9, you’ll have a digit to carry to the next column. On the abacus, you’d have a number set already – then, to add another number to it, you could work from the right, adding the number to the digit and increasing the next column along if you need a carry.
Subtraction is more difficult – on paper, subtracting two numbers sometimes requires you to ‘borrow’ some of the value of the next column. One way to achieve subtraction on an abacus is using the method of complements: substituting a digit for its value when you subtract it from 9. For example, 0 can be substituted for 9, 1 for 8, 2 for 7 and so on. This is sometimes called ‘9’s complement’, and is a way to subtract things while only performing addition. Subtracting two numbers can be achieved by taking the complement of the larger number, adding them together, and taking the complement of the result.
For example, if you wanted to calculate 832 – 491, you can take the complement of 832 (which is 167), add it to 491 (167 + 491 = 658) and then find the complement of 658 (which is 341, the answer we needed). If you’d like to take a minute to convince yourself why this works, please do.
Similar methods exist to perform multiplication and division digit-by-digit, which can be used with an abacus. It’s also possible to use an abacus to find square and cube roots.
In 1946 a competition was held to determine which was quicker at calculating – a human using a soroban, or one using an electric calculator. Each were challenged to add together 50 large numbers, and perform similar feats of subtraction, multiplication and division. The soroban outperformed the electric calculator on addition, subtraction, division and on problems combining multiple operations – the only category in which the electric calculator prevailed was in multiplication.
Another advantage of the abacus was that early calculators were limited to 8-10 digits, and a soroban can handle as many digits as you have columns of beads. Since the only calculations you can’t really do on an abacus are those of higher complexity, such as trigonometric functions, it was not considered to be superseded by the calculator as a tool until relatively recently.
Adding up to a win
Many people use abacus calculation as a way to improve their general mental agility – abacists (the term for people who use an abacus) have been shown to have improved memory, concentration, speed of thought and mental capacity. In parts of East Asia, there are soroban competitions, where participants compete to solve calculations faster and faster. Schools in Japan run after-school abacus clubs, where students train and practice for competitions.

The competitions even include mental calculation rounds, in which participants visualise an imaginary abacus and use it to perform calculations – adding together a series of numbers flashed up briefly on a screen, and only writing down the final answer. In 2012, a world record was set for the correct addition of 15 three-digit numbers each shown on a screen for only 1.7 seconds each, beating the previous record of 1.8 seconds.
Abacuses similar in design to a soroban, with a piece of rubber or fabric on the back to stop the beads from sliding down, can be used by visually impaired people to perform calculations by feeling the position of the beads. While talking calculators can be used for more complicated calculations, the abacus performs the same function as a pencil and paper would – allowing people to perform digit-wise calculations and keep track of what they’re doing.
As a symbol of mathematics and computation, the abacus is a simple form of technology, integrated with mathematical ideas, which has revolutionised the way people interact with mathematics. The IMU Abacus Medal will continue to recognise the achievements of those who are creating and working with the modern equivalent of the abacus – using hardware and software, combined with the power mathematical ideas, to change the world.


No, IMU gave no reason publicly why they removed the name of Rolf Nevanlinna from the prize and the medal.
I suggest you research first, and then write – just my two cents.