One of many ways of characterizing the typical HLF attendee is probably the fact that, when faced with a blog article series called “6 out of 200“, their first reaction is not likely to be “oh, cool, let me read about six of the other 200 young researchers attending the meeting”. Much more likely is “Hm. 6 out of 200. That’s a binomial coefficient.”

If you knew their next thought, you could probably start differentiating between the different types of HLF attendee.

Really, really pure mathematicians (or so a pertinent joke goes) would probably think “6 out of 200? The problem is solvable, and the solution is unique”, and leave it at that.

If you are slightly more practically inclined, your brain will offer up the definition for the binomial coefficient,

so in this case

which, written out is

– and again, this is an opportunity to tell the more purely minded from those inclined towards the applications. The latter will start calculating, or at least think about the most efficient way to calculate this. My first thought when seeing this expression is, admittedly, “Tell me again why we can always be sure the result of a fraction like the one on the right-hand side is an integer?”

The simplest way of seeing that for binomial coefficients is, of course, to show that n over k is indeed the number of possibilities to choose k elements out of a set containing n elements. But I’m thinking about a somewhat more general case. In the numerator we have six consecutive numbers, so exactly one of them must be divisible by six. Does this generalize to the remaining factors in the denominator? In other words: Is the product of k consecutive numbers always divisible by k! or not?

I’ve played around with this a bit, but didn’t find a proof right away, so I turned to my computer for help, writing a simple perl script to look for counterexamples. The script found counterexamples, but they didn’t check out when I re-calculated the first of them by hand. Apparently, I’d run into the limitations of numerics – the numbers obviously became quite large, and perl couldn’t keep them stored with sufficient accuracy to perform the simple calculation of dividing one by the other correctly.

So can any reader come up with a simple proof – or a counter-example?

Back to our original question,

so we would need to enlist the entire population of the Earth to get the whole interview series. (And even then, they would have to work in parallel.)

Another computer-y question: Would it have helped if we had asked a search engine?

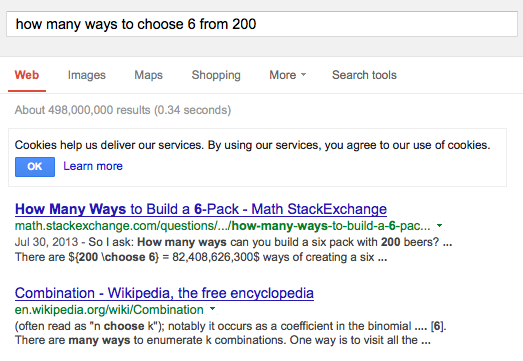
Let’s start with Google: “How many ways to choose 6 out of 200”?

As always, Google does its best to amuse us as it attempts to second-guess what we’re asking:

A TV series, a sartorial cry for help, so far, so good. I’ll refrain from speculating at what point in the date the couple takes a time-out to google number 3. And I was going to make a snarky comments about the inability to spell “horse”, but apparently that word, which I didn’t know before, is a game “With Howrse, breed horses or ponies, and discover the responsibilities of managing an equestrian center!”

I just had to follow up on “how many days until”, and apparently number 1 is “until christmas” and number 2 is “until my birthday”. Which, in the days of PRISM, is probably not as stupid a question to ask a search engine as it once was.

Turns out there are only two ways to amend the constitution, if you restrict yourself to the number of procedures, that is.

I don’t know about the egg-cooking, but “How many ways to tie a tie” actually is a cool mathematical problem related to random walks on a triangular lattice, as shown in the book The 85 Ways to Tie a Tie (OK, sorry, forgot to put “spoiler alert”).

Almost there.

Bagels!

Not a direct answer by Google, but the first link gives the right answer.

The next contestant: Bing.

Apparently, Bing is localized, as a number of completions are in German. It doesn’t know (or pretends it doesn’t know) that I do not care for horses all that very much. A good sign, I guess.

I was a bit disappointed, frankly, that Google didn’t assume I wanted to know about the roads to walk down.

From this point on, Bing pointedly refrains from trying to second-guess me.

…and the list goes on, but at least on the first page, there is no answer to the question I posed. Lots of answers to vaguely similar-looking questions, though. That’s a clear fail, Bing!

Then, there’s Wolfram Alpha. It doesn’t attempt to auto-complete, and it clearly understands the question:

I didn’t find any way of telling it I wanted an exact, answer, though. Just adding “Exact answer, please!” didn’t change anything.

Google for the win, in this case!

So – lessons learned?

Mathematics is almost as powerful at diverting you than the Internet (I spent more time on trying to find the proof I mentioned above than playing with the search engines).

and

Boy, are we far away from intelligent computers, and intelligent search engines.

and

Simple mathematics, and numerical problems, and computer science, are everywhere.

Also, if you find the time, you might want to read the actual blog posts in the “6 out of 200” series – here‘s the first one, but apparently, the series doesn’t have a common tag (yet).