In my earlier post on the cost of reaching geostationary orbit from the launch orbit that was achieved by Ariane flight VA241, I stated that the approximate impulsive cost I have obtained for the Al Yah 3 satellite amounts to 2168 m/s. This value is of course subject to certain assumptions, which I also stated. Now if the satellite doesn’t have sufficient propellant to provide that delta-v then what one really wants to know is what final orbit can be reached with the insufficient amount of delta-v that is available.

(Author’s Note: All I am providing here is my personal opinion and some results of mathematical calculations I have performed myself based on publicly available information. These results could have been obtained by anyone with a background in orbital dynamics. Nothing I write here shall be construed as reflecting the official position of my employer. Essentially, what we have here is a mathematical problem: A spacecraft is in a given initial orbit and shall reach a certain target orbit B with a certain amount of delta-v. What I am presenting is a mathematical solution to this problem that I have identified. Now, back to the blog article …)

And that’s where things turn complicated. I have set equality constraints on the final semi-major axis, eccentricity and inclination. The semi-major axis shall be the geostationary value, the eccentricity and the inclination shall be zero. I.e., the nominal geostationary orbit. To get there from the current orbit, a certain minimum delta-v is required, assuming the three-burn strategy I specified. If there are more manoeuvres and especially if there are long waiting times with natural perturbations integrated in the sequence, then a much different solution may be found.

But assuming that the strategy remains as specified, and constraining the delta-v to less than the minimum I identified, something’s gotta give. There could be a compromise on the inclination or on the semi-major axis and inclination, with the rationale that the spacecraft’s ion propulsion is used to reach the final orbit. One could accept some difference in all of the parameters. But there, there might be an infinite number of solutions, each reachable with the same delta-v.

If all the solutions are mapped into a diagram that shows the entire solution space, you end up with something that is called a Pareto front. A Pareto front is a sharply delineated region for which solutions can be found that comply with the hard constraint (here the fact that there is insufficient propellant to reach the target orbit) while violating each of the soft constraints to different extents: Some of the solution points pertain to cases where the final inclination is close to zero but the mean radius is far off and the orbit is still quite eccentric, others may pertain to an almost circular final orbit with a period close to 24 hours but with an inclination that is quite large,while other solution points lie somewhere in between.

It may sound complicated, but really, a Pareto front is akin to situations we face in everyday life, where we are confronted  with a problem that can be solved in a lot of different ways, none of which is completely to our liking, and we have to find the one solution that hurts the least.

Out of this infinite set, one target must be selected. This selection will most likely be based on many things that I don’t know, mostly the characteristics of the ion propulsion system and the thermal and power constraints applying to the Al Yah 3 satellite. Even presenting a reasonably well-populated Pareto front entails far more effort that I am willing to invest, especially as I am investing my own free time and nobody is paying me to do this. If I get invited to the Emirates with a free stay at the Burj al Arab then I’ll gladly crunch  more numbers.

But not before. So all I can offer now is a set of exemplary results, i.e., excerpts from the respective Pareto fronts, for a series of allowed Delta-v limits.

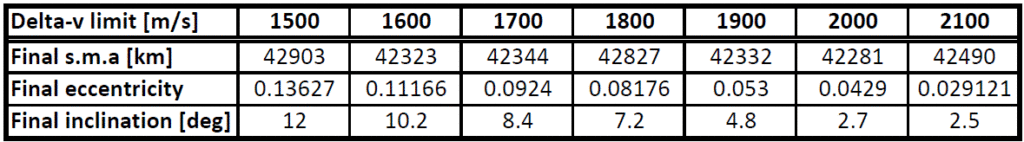
Unsurprisingly, if the delta-v limit is far from the identified minimum, there will be a large residual inclination and eccentricity. Only when the available delta-v reaches or exceeds 2000 m/s will the final inclination be in the low single digits range. All now depends on the available conventional delta-v margins … and the capabilities of the ion propulsion system. For the latter, the issue is not only propellant mass but also on the thrust level and the resulting acceleration.

An ion propulsion system that is used for station keeping runs while Al Yah 3’s payload is operating, so it must share the power input from the solar arrays with the transponders. Typically, such an ion propulsion system delivers only limited thrust, which is enough for stationkeeping, but which would make orbital transfer operations a very lengthy and tedious process.